Fractional Integration and Cointegration. Theoretical Advances in Univariate and Multivariate Models
Applications in Economics, Finance and Other Areas with Time Series
This Project focuses on the study of time series. More in particular, it will be centred on fractional integration and cointegration, and, in general, on long memory processes, also named strongly dependent processes. The project has two differentiated sections, one theoretical and the other one empirical.
is related with the univariate and multivariate extensions of fractionally integrated models to non-linear structures of stochastic nature, and with spectral density functions that show a singularity or pole at the zero frequency.

In a similar way, we will also study in the project the cases where the spectrum displays one or more poles or singularities at frequencies away from zero, allowing for the presence of structural breaks at unknown periods of time.
We will also examine multiple cyclical structures with the number of cycles being endogenously determine by the model itself from the data.

Multivariate Fractional Vector AutoRegressive (FVAR) models will also be examined in the paper, with series displaying different orders of integration.
This will allow us study simultaneously series displaying different degrees of persistence. We will propose test statistics for testing equality in the orders of integration as a preliminary step for the analysis of fractional cointegrated models (FCVAR).
In the project we will also develop the codes for the application of the theoretical models above presented and these codes will be employed in a number of time series, covering many areas such as macroeconomics, finance, cryptocurrencies, development economics, tourism, environmental studies and climate change.

In this empirical context we will study theories such as the Fisher hypothesis, the Purchasing Power Parity, hysteresis in unemployment, the efficiency in the stock markets, etc. using the econometric techniques proposed in the project.

We will also examine the degree of persistence in time series such as inflation and unemployment, investigating if such degree of persistence has changed across time.
In multivariate contexts, we will look at relationships among variables such as prices and wages, short run and long run interest rates, stock market prices and dividends, or consumption and income, etc.
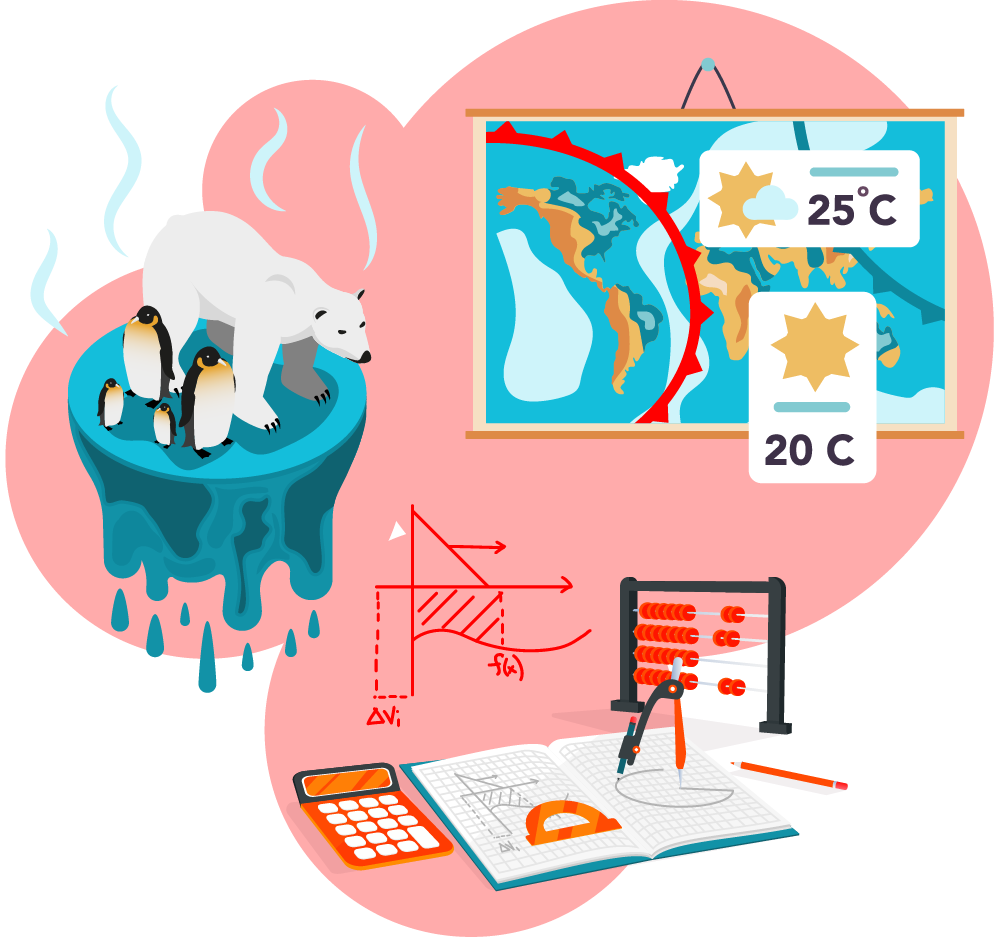
In relation with environmental studies, we will look at the presence of linear and nonlinear trends in pollution agents such as Particular Matters or CO2, and dealing with climate we will examine hypotheses such as the Artic Amplification or the Global Warming.
Our team
PRINCIPAL INVESTIGATOR
RESEARCH TEAM
Ernesto María Gavassa Pérez (School of Economics)
Itzel De Haro López (Navarra Center for International Development)
PID2023-149516NB-I00


